1. Complex projective plane serves as a canvas

Next: 1. Pure states as Up: 4. Notes Previous: 4. Notes

1. Complex projective plane serves as a canvas

Pure states of the quantum spin (we are discussing spin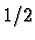

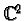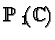here) are described by unit vectors in our Hilbert space $\Cset^2$ . But, as it is standard in quantum theory, proportional vectors describe the same state - the overall phase of the vector has no physical significance. Therefore, in geometrical terms, the set of all pure states is nothing but the projective complex space $\mathbb {P}_1(\Cset)$ which happens to be the same as the sphere

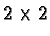That is why the fractal pattern, in our case, is being drawn on a spherical canvas. There is, however, another possible interpretation of the same algorithm. It is well know that there is an intrinsic relation between Minkowski space and the space of $2\times 2$ complex Hermitean matrices. In coordinates the map is given by

$\begin{displaymath} p=\{p^\mu\}\mapsto \FMslash{p}\doteq p^\mu\sigma_\mu = \pmatrix{p^0+p^3,&p^1-{\mathrm{i}}p^2\cr p^1+{\mathrm{i}}p^2,&p^0-p^3} \end{displaymath}$

(18)

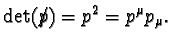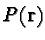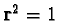so that $\mathrm{det}(\FMslash{p})=p^2=p^\mu p_\mu .$ In particular our projection operators $P({\bf r})$ , ${\bf r}^2=1$ correspond to null directions. In other words our canvas, the sphere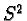

, can be also thought of as the projective light cone - the space of light directions. Quantum jumps would then correspond to sudden changes of directions of light or light-like entities. The formula (

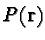) can be easily generalized for $P({\bf r})$ being a generic Hermitean matrix (thus representing a four-vector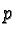

of space- or time-like character as well. However there is no such generalization for the formula (

), so that the probabilities would have to be assigned equal, and a physical interpretation, even tentative one, is missing in such a case.

Next: 1. Pure states as Up: 4. Notes Previous: 4. Notes

2002-04-11