

Next: 2. Fuzzy projections
Up: 4. Notes
Previous: 1. Complex projective plane
For a spin 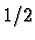 quantum system pure quantum states are uniquely
described by projection operators
quantum system pure quantum states are uniquely
described by projection operators 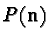 , where
, where 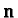 is a unit
length direction vector, starting at the origin, and ending on one
of the points of the unit sphere. All pure states are of this
form. Indeed, every orthogonal projection, except of the two
trivial ones:
is a unit
length direction vector, starting at the origin, and ending on one
of the points of the unit sphere. All pure states are of this
form. Indeed, every orthogonal projection, except of the two
trivial ones:  and
and  are of the form
are of the form 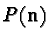 for some
for some 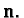
2002-04-11