Introduction

Time plays a peculiar role in Quantum Mechanics. It differs from other physical quantities like position or momentum. When discussing position a dialogue may look like this:

SP: What is the position?
SG: Position of what?
SP: Of the particle.
SG: When?
SP: At t=t1.
SG: The answer depends on how you are going to measure this position. Are you sure you have detectors put everywhere that interact with the particle only during the time interval (t-dt,t+dt) and not before?

When talking about time we will have something like this:

SP: What is time?
SG: Time of what?
SP: Time of a particle.
SG: Time of your particle doing what?
SP: Time of my particle leaving the box where it was trapped. Or time at which my particle enters the box.
SG: Well, it depends on the box and it depends on the method you want to apply to ascertain that the event has happened.
SP: Why can't we simply put clocks everywhere, as it is common in discussions of special relativity? And let these clocks note the time at which the particle passes them?
SG: Putting clocks disturbs the system. The more clocks you put - the more you disturb. If you put them everywhere - you force wave packet reductions a`la GRW. If you increase their time resolution more and more - you increase the frequency of reductions. When the clocks have infinite resolutions - then the particle stops moving - this is the Quantum Zeno effect [2].
SP: I do not believe these wave packet reductions. Zeh published a convincing paper whose title tells its content: "There are no quantum jumps nor there are particles" [3], and Ballentine [4,5], proved that the projection postulate is wrong.
SG I remember these papers. They had provocative titles...
SV. First of all Ballentine did not claim that the projection postulate is wrong. He said that if incorrectly applied - then it leads to incorrect results. And indeed he showed how incorrect application of the projection postulate to the particle tracks in a cloud chamber leads to inconsistency. What he said is this: "According to the projection postulate, a position measurement should "collapse" the state to a position eigenstate, which is spherically symmetric and would spread in all directions, and so there would be no tendency to subsequently ionize only atoms that lie in the direction of the incident momentum. An approximate position measurement would similarly yield a spherically symmetric wave packet, so the explanation fails." This is exactly what he said. And this is correct. This shows how careful one has to be with the projection postulate. If the projection postulate is understood as operating with an operator on a state vector: , then the argument does not apply. Thus a correct application would be to multiply the moving Gaussian of the particle, something like:

which is spherically symmetric, but only up to the phase, by a static Gaussian modelling a detector localized around a:

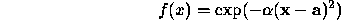
The result is again a moving Gaussian. And in fact, such a projection postulate is not a postulate at all. It can be derived from the simplest possible Liouville equation.
SP: Has this "correct", as you claim, cloud chamber model been published? Have its predictions been experimentally verified?
SV: A general theory of coupling between quantum system and a classical one is now rather well understood [6]. The cloud chamber model has been published quite recently, you can take a look at [7,8]. Belavkin and Melsheimer [9] tried to derive somewhat similar result from a pure unitary evolution, but I am not able to say what assumptions they used, what approximations they made and what exactly are their results.
SP: Hasn't the problem been solved long ago in the classical paper by Mott [10]?
SV Mott did not even attempt to derive the timing of the tracks. In the cloud chamber model of Refs. [7,8], that I understand rather well, because I participated in its construction, it is interesting that the detectors -- even if they do not "click" -- influence the continuous evolution of the wave packet between reductions. They leave a kind of a "shadow". This is another case of a "interaction-free" experiment discussed by Dicke [11,12], and then by Elitzur and Vaidman [13] in their "bomb--test" allegory, and also by Kwiat, Weinfurter, Herzog and Zeilinger [14]. The shadowing effect predicted by EEQT may be tested experimentally. I believe it will find many applications in the future, and I hope these will be not only the military ones! Yet we must now not digress upon this particular topic since you are waiting to hear what I think about the problem of time in quantum theory. We already know that "time" must be "time of something". Time of something that happens. Time of some event. But in quantum theory events are not simply space-time events as it is in relativity. Quantum theory is specific in the sense that there are no events unless there is something external to the quantum system that "causes" these events. And this something external must not be just another quantum system. If it is just another quantum system - then nothing happens, only the state vector continuously evolves in parameter time.
SP But is it not so that there are no sharp events? Nothing is sharp, nothing really sudden. All is continuous. All is approximate.
SG How nothing is sharp, do we not register "clicks" when detecting particles?
SP I do not know what clicks are you talking about ...
SG How you don't know? Ask the experimentalist.
SP I am an experimentalist!
SV The problem you are discussing is not an easy one to answer. I pondered on it many times, but did not arrive at a clear conclusion. Nevertheless something can be said with certainty. First of all you both agree that in physics we always have to deal with idealizations. For instance one can argue that there are no real numbers, that the only, so to say, experimental numbers are the natural numbers. Or at most rational numbers. But real numbers proved to be useful and today we are open to both possibilities: of a completely discrete world, and of a continuous one. Perhaps there is also a third possibility: of a fuzzy world. Similarly there are different options for modeling the events. One can rightly argue that they are never sharp. But do they happen or not? Do we need counting them? Do we need a theory that describes these counts? We do. So, what to do? We have no other choice but to try different mathematical models and to see which of them better fit the experiment, better fit the rest of our knowledge, better explain what makes Nature tick. In the cloud chamber model that we were talking about just a while ago the events are unsharp in space but they are sharp in time. And the model works quite well. However, if you try to work out a relativistic cloud chamber model, then you see that the events must be also smeared out in the coordinate time. Nevertheless they can still be sharp in a different "time", called "proper time" after Fock and Schwinger. If time allows I will tell you more about this relativistic theory, but now let us agree that in a nonrelativistic theory sharp localization of events in time does not contradict any known principles. We will remember at the same time that we are dealing here with yet another idealization that is good as long as it works for us. And we must not hesitate to abandon it the moment it starts to lead us astray. The principal idea of EEQT is the same as that expressed in a recent paper by Haag [17]. Let me quote only this: "... we come almost unavoidably to an evolutionary picture of physics. There is an evolving pattern of events with causal links connecting them. At any stage the `past' consists of the part which has been realized, the `future' is open and allows possibilities of new events ..."
SG Let me interrupt you. Perhaps we should remember what Bohr was telling us. Bohr insisted that the apparatus has to be described in terms of classical physics; this point of view is a common--place for experimental physicists. Indeed any experimental article observes this rule. This principle of Bohr is not in any way a contradiction but simply the recognition of the fact that any physical theory is always the expression of an approximation and an idealization. Physics is always a little bit false. Epistemology must also play role in the labs. Physics is a system of analogies and metaphors. But these metaphors are helping us to understand how Nature does what it does.
SP I agree with this. So what is your proposal? How to describe time of events in a nonrelativistic quantum theory? Does one first have to learn EEQT - your "Event Enhanced Quantum Theory" that you are so proud of? I know many theoretical physicists dislike your explicit introduction of a classical system. They prefer to keep everything classical in the background. Never put it into the equations.
SV Here we have a particularly lucky situation. For this particular purpose of discussing time of events it is not necessary to learn EEQT. It is possible to describe time observation with simple rules. This is normal in standard quantum mechanics. You are told the rules, and you are told that they work. So you believe them and you are happy that you were told them. In EEQT Schrödinger's evolution and reduction of the wave function appear as special cases of a single law of motion which governs all systems equally. EEQT is one of the few approaches that allow you to derive quantum mechanical postulates and to see that these postulates reflect only part of the truth. Here, when discussing time of events we do not need the full predictive power of EEQT. This is so because after an event has been registered the experiment is over. We are not interested here in what happens to our system after that. Therefore we need not to speak about jumps and wave packet reductions. It is only if you want to derive the postulates for time measurements, only then you will have to look at EEQT. But instead of deriving the rules, it is even better to see if they give experimentally valid predictions. We know too many cases where good formulas were produced by doubtful methods and bad formulas with seemingly good ones. Using the right tool makes the job easier.
SP I become impatient to see your postulates, and to see if I can accept them as reasonable even before any experimental testing. Only if I see that they are reasonable, only then I will have any motivation to see whether they really be derived from EEQT, or perhaps in some other way.